Re: 2012 HSC MX2 Marathon
 = p(x)=\sum_{k=0}^{2n} \frac{x^k}{k!})
Expanding, we get:
 = 1 + x + \frac{x^2}{2!} + \frac{x^4}{4!} + .... + \frac{x^2^n}{2n!})
Thus for P(x) to be zero, the terms after 1 must add up to -1. Hence:
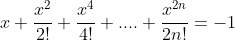
BUT, there is no real value of x for which the above sum can possibly equal to -1. Therefore, there are no real roots for P(x).
EDIT: say. if you put x = -2, the value of the sum will be a limit that approaches -1, but never reaches -1. this would be the same for all x = m, where the sum will approach m + 1.
I don't know if this is right, but I'll give it a shot.Might as well bring this back to life.
Prove that the polynomial
has no real roots. (Where n is a non-negative integer.)
Expanding, we get:
Thus for P(x) to be zero, the terms after 1 must add up to -1. Hence:
BUT, there is no real value of x for which the above sum can possibly equal to -1. Therefore, there are no real roots for P(x).
EDIT: say. if you put x = -2, the value of the sum will be a limit that approaches -1, but never reaches -1. this would be the same for all x = m, where the sum will approach m + 1.
Last edited:
