4U Revising Game (1 Viewer)
- Thread starter ronnknee
- Start date
GUSSSSSSSSSSSSS
Active Member
HAHAHAHAThat does look about right....
I dont have the answers btw
..........i mite be able to get a hold of them
Prove that for a > b > 0:

nice solution
gurmies
Drover
New MX2 Game
Hey guys, with trial exams looming up ahead, I thought it would be a good idea to revive the MX2 game. Questions should be mainly taken from trial exams (it would be a good idea to quote which school and year) and to make things interesting, try to make them difficult (although this is dependent on perception). Furthermore, to keep things interesting for those who weren't the first to answer the question, add spoiler tags (spoiler) and (/spoiler), but using [] instead. One last thing: If a question has been answered, but you believe you have an alternative method, please post it (irrespective of how strange it may be) because exposure to different approaches will expand our own ways of thinking about problems. To start things off:
Sydney Technical High School 1996
Question 8, Part b)
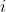Explain \,\, why \,\, (6+\sqrt{35})^{1980}+(6-\sqrt{35})^{1980} \,\, is \,\, an \,\, even \,\, number \\\\ ii)Show \,\, that \,\, 0<6-\sqrt{35}<0.1 \\\\ iii)Hence \,\, or \,\, otherwise \,\, prove \,\, that \,\, the \,\, first \,\, thousand \,\, digits \,\, after \,\, the \,\, decimal \,\, point \,\, in \,\, the \,\, value \,\, of \,\, (6+\sqrt{35})^{1980} \,\, are \,\, all \,\, 9's.)
Hey guys, with trial exams looming up ahead, I thought it would be a good idea to revive the MX2 game. Questions should be mainly taken from trial exams (it would be a good idea to quote which school and year) and to make things interesting, try to make them difficult (although this is dependent on perception). Furthermore, to keep things interesting for those who weren't the first to answer the question, add spoiler tags (spoiler) and (/spoiler), but using [] instead. One last thing: If a question has been answered, but you believe you have an alternative method, please post it (irrespective of how strange it may be) because exposure to different approaches will expand our own ways of thinking about problems. To start things off:
Sydney Technical High School 1996
Question 8, Part b)
Last edited:
untouchablecuz
Active Member
- Joined
- Mar 25, 2008
- Messages
- 1,693
- Gender
- Male
- HSC
- 2009
Re: New MX2 Game
nearly done the last bit
Another Q:
Sydney Tech 2001, 8 c)
i) If x1 > 1 and x2 > 2 show that x1 + x2 > sqrt(x1x2)
ii) Use the principle of mathematical induction to show that, for n ≥ 2, if xj > 1 where j = 1, 2, 3, . . . , n then ln(x1+x2+· · ·+xn) > [1/(2n−1)](lnx1 +lnx2+· · ·+lnxn).
(the numeral or pronumeral after "x" is in subscript xj, xn, x1 etc)
nearly done the last bit
Another Q:
Sydney Tech 2001, 8 c)
i) If x1 > 1 and x2 > 2 show that x1 + x2 > sqrt(x1x2)
ii) Use the principle of mathematical induction to show that, for n ≥ 2, if xj > 1 where j = 1, 2, 3, . . . , n then ln(x1+x2+· · ·+xn) > [1/(2n−1)](lnx1 +lnx2+· · ·+lnxn).
(the numeral or pronumeral after "x" is in subscript xj, xn, x1 etc)
Re: New MX2 Game
(i) [maths](\sqrt{x_1}-\sqrt{x_2})^2\geq 0\\x_1+x_2\geq 2\sqrt{x_1x_2}\geq \sqrt{x_1x_2}[/maths]
(ii) [maths]$for n=2,$\\\ln(x_1+x_2)>\ln(\sqrt{x_1x_2})\\=\frac{1}{2}\ln(x_1x_2)\\>\frac{1}{2(2)-1}\ln(x_1x_2)\\\\$assume n=k is true,\\for n=k+1,$\\\ln((x_1+x_2+...+x_k)+x_{k+1})\\=\ln(x_1+x_2+...+x_k)+\ln(x_{k+1})\\>\frac{1}{2n-1}(\ln x_1+\ln x_2+...+\ln x_n)+\ln( x_{k+1})\\=\frac{1}{2n-1}(\ln x_1+\ln x_2+...+\ln x_n+(2n-1)\ln x_{k+1})\\>\frac{1}{2n-1}(\ln x_1+\ln x_2+...+\ln x_n+\ln x_{k+1})forn\geq 2[/maths]
for the last part of 1st question, cant you just say 0<[6-sqrt(35)]^1980<(0.1)^1980 so [6-sqrt(35)]^1980 must be all 0's for the first 1979 decimal places and since [6-sqrt(35)]^1980 + [6+sqrt(35)]^1980 add up to an even number (a whole number), then [6+sqrt(35)]^1980 must be all 9's for first thousand decimal places.
(i) [maths](\sqrt{x_1}-\sqrt{x_2})^2\geq 0\\x_1+x_2\geq 2\sqrt{x_1x_2}\geq \sqrt{x_1x_2}[/maths]
(ii) [maths]$for n=2,$\\\ln(x_1+x_2)>\ln(\sqrt{x_1x_2})\\=\frac{1}{2}\ln(x_1x_2)\\>\frac{1}{2(2)-1}\ln(x_1x_2)\\\\$assume n=k is true,\\for n=k+1,$\\\ln((x_1+x_2+...+x_k)+x_{k+1})\\=\ln(x_1+x_2+...+x_k)+\ln(x_{k+1})\\>\frac{1}{2n-1}(\ln x_1+\ln x_2+...+\ln x_n)+\ln( x_{k+1})\\=\frac{1}{2n-1}(\ln x_1+\ln x_2+...+\ln x_n+(2n-1)\ln x_{k+1})\\>\frac{1}{2n-1}(\ln x_1+\ln x_2+...+\ln x_n+\ln x_{k+1})forn\geq 2[/maths]
for the last part of 1st question, cant you just say 0<[6-sqrt(35)]^1980<(0.1)^1980 so [6-sqrt(35)]^1980 must be all 0's for the first 1979 decimal places and since [6-sqrt(35)]^1980 + [6+sqrt(35)]^1980 add up to an even number (a whole number), then [6+sqrt(35)]^1980 must be all 9's for first thousand decimal places.
untouchablecuz
Active Member
- Joined
- Mar 25, 2008
- Messages
- 1,693
- Gender
- Male
- HSC
- 2009
Re: New MX2 Game
this part doesnt follow; try begining with the use of the result a + b > sqrt(ab) from i)
[maths]ln((x_1+x_2+...+x_k)+x_{k+1})\\=\ln(x_1+x_2+...+x_k)+\ln(x_{k+1})[/maths](i) [maths](\sqrt{x_1}-\sqrt{x_2})^2\geq 0\\x_1+x_2\geq 2\sqrt{x_1x_2}\geq \sqrt{x_1x_2}[/maths]
(ii) [maths]$for n=2,$\\\ln(x_1+x_2)>\ln(\sqrt{x_1x_2})\\=\frac{1}{2}\ln(x_1x_2)\\>\frac{1}{2(2)-1}\ln(x_1x_2)\\\\$assume n=k is true,\\for n=k+1,$\\\ln((x_1+x_2+...+x_k)+x_{k+1})\\=\ln(x_1+x_2+...+x_k)+\ln(x_{k+1})\\>\frac{1}{2n-1}(\ln x_1+\ln x_2+...+\ln x_n)+\ln( x_{k+1})\\=\frac{1}{2n-1}(\ln x_1+\ln x_2+...+\ln x_n+(2n-1)\ln x_{k+1})\\>\frac{1}{2n-1}(\ln x_1+\ln x_2+...+\ln x_n+\ln x_{k+1})forn\geq 2[/maths]
for the last part of 1st question, cant you just say 0<[6-sqrt(35)]^1980<(0.1)^1980 so [6-sqrt(35)]^1980 must be all 0's for the first 1979 decimal places and since [6-sqrt(35)]^1980 + [6+sqrt(35)]^1980 add up to an even number (a whole number), then [6+sqrt(35)]^1980 must be all 9's for first thousand decimal places.
this part doesnt follow; try begining with the use of the result a + b > sqrt(ab) from i)
Last edited:
Re: New MX2 Game
woops, thanks for the correction. 2nd attempt:
[maths](x_1+x_2+...+x_k)+x_{k+1}>\sqrt{(x_1+x_2+...+x_k)x_{k+1}}\\\ln(x_1+x_2+...+x_k+x_{k+1})\\>\frac{1}{2}(\ln(x_1+x_2+...+x_k)+\ln(x_{k+1}))\\>\frac{1}{2}(\frac{1}{2k-1}(\ln x_1+\ln x_2+...+\ln x_k+(2k-1)\ln x_{k+1}))\\>\frac{1}{4k-2}(\ln x_1+\ln x_2+...+\ln x_k+\ln x_{k+1})\\>\frac{1}{2k+1}(\ln x_1+\ln x_2+...+\ln x_k+\ln x_{k+1})forn>2\\=\frac{1}{2(k+1)-1}(\ln x_1+\ln x_2+...+\ln x_k+\ln x_{k+1})[/maths]
woops, thanks for the correction. 2nd attempt:
[maths](x_1+x_2+...+x_k)+x_{k+1}>\sqrt{(x_1+x_2+...+x_k)x_{k+1}}\\\ln(x_1+x_2+...+x_k+x_{k+1})\\>\frac{1}{2}(\ln(x_1+x_2+...+x_k)+\ln(x_{k+1}))\\>\frac{1}{2}(\frac{1}{2k-1}(\ln x_1+\ln x_2+...+\ln x_k+(2k-1)\ln x_{k+1}))\\>\frac{1}{4k-2}(\ln x_1+\ln x_2+...+\ln x_k+\ln x_{k+1})\\>\frac{1}{2k+1}(\ln x_1+\ln x_2+...+\ln x_k+\ln x_{k+1})forn>2\\=\frac{1}{2(k+1)-1}(\ln x_1+\ln x_2+...+\ln x_k+\ln x_{k+1})[/maths]
Last edited:
untouchablecuz
Active Member
- Joined
- Mar 25, 2008
- Messages
- 1,693
- Gender
- Male
- HSC
- 2009
Re: New MX2 Game
with your Q, i used sum to products, i end up with every term having a 2cos(-alpha/2) as a factor but thats where im stuck
niceewoops, thanks for the correction. 2nd attempt:
[maths](x_1+x_2+...+x_k)+x_{k+1}>\sqrt{(x_1+x_2+...+x_k)x_{k+1}}\\\ln(x_1+x_2+...+x_k+x_{k+1})\\>\frac{1}{2}(\ln(x_1+x_2+...+x_k)+\ln(x_{k+1}))\\>\frac{1}{2}(\frac{1}{2k-1}(\ln x_1+\ln x_2+...+\ln x_k+(2k-1)\ln x_{k+1}))\\>\frac{1}{4k-2}(\ln x_1+\ln x_2+...+\ln x_k+\ln x_{k+1})\\>\frac{1}{2k+1}(\ln x_1+\ln x_2+...+\ln x_k+\ln x_{k+1})forn>2\\=\frac{1}{2(k+1)-1}(\ln x_1+\ln x_2+...+\ln x_k+\ln x_{k+1})[/maths]
with your Q, i used sum to products, i end up with every term having a 2cos(-alpha/2) as a factor but thats where im stuck
Re: New MX2 Game
Nice question on sums-to-products from the extension part of yr12 Cambridge 3unit book.
lol, all these MX2/MX1 game's are doomed to fail.Hey guys, with trial exams looming up ahead, I thought it would be a good idea to revive the MX2 game. Questions should be mainly taken from trial exams (it would be a good idea to quote which school and year) and to make things interesting, try to make them difficult (although this is dependent on perception). Furthermore, to keep things interesting for those who weren't the first to answer the question, add spoiler tags (spoiler) and (/spoiler), but using [] instead. One last thing: If a question has been answered, but you believe you have an alternative method, please post it (irrespective of how strange it may be) because exposure to different approaches will expand our own ways of thinking about problems. To start things off:
Sydney Technical High School 1996
Question 8, Part b)
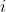Explain \,\, why \,\, (6+\sqrt{35})^{1980}+(6-\sqrt{35})^{1980} \,\, is \,\, an \,\, even \,\, number \\\\ ii)Show \,\, that \,\, 0<6-\sqrt{35}<0.1 \\\\ iii)Hence \,\, or \,\, otherwise \,\, prove \,\, that \,\, the \,\, first \,\, thousand \,\, digits \,\, after \,\, the \,\, decimal \,\, point \,\, in \,\, the \,\, value \,\, of \,\, (6+\sqrt{35})^{1980} \,\, are \,\, all \,\, 9's.)
Nice question on sums-to-products from the extension part of yr12 Cambridge 3unit book.
gurmies
Drover
Re: New MX2 Game
Yeah man, always a lack of participation! Perhaps people are too engrossed in their trials study =Dlol, all these MX2/MX1 game's are doomed to fail.
Nice question on sums-to-products from the extension part of yr12 Cambridge 3unit book.
x.Exhaust.x
Retired Member
Re: New MX2 Game
In other words, C B F.
In other words, C B F.
clintmyster
Prophet 9 FTW
- Joined
- Nov 12, 2007
- Messages
- 1,067
- Gender
- Male
- HSC
- 2009
- Uni Grad
- 2015
Re: New MX2 Game
so you scan like I do =P
so you scan like I do =P
Revacious
o-o
- Joined
- Aug 6, 2008
- Messages
- 140
- Gender
- Undisclosed
- HSC
- N/A
Re: New MX2 Game

I shat myself when i saw this question, i mean, it was a diagram that took the whole damn page up.
It wasn't too bad when you did part abcd, but for the fun of it, let's skip to the last part =)
Find the motherfucking volume of that motherfucking solid. (SB THSC 2004)

I shat myself when i saw this question, i mean, it was a diagram that took the whole damn page up.
It wasn't too bad when you did part abcd, but for the fun of it, let's skip to the last part =)
Find the motherfucking volume of that motherfucking solid. (SB THSC 2004)
