THANKS A LOT!The amount of zeros a number ends in is the amount of 10s that are in the prime factorization. Now 10 = 2 x 5. And so when we prime factorize 2008! we need to count the amount of 2s and the amount of 5s.
Now there is clearly going to be more 2s than 5s, so to determine how many factors of 10 there are in the prime factorization of 2008! we must only find how many 5s are in the prime factorization.
Now clearly finding the prime factorization of 2008! would be a torturous task, so we will "look" at each term of 2008! ie 1, 2, 3, 4, 5, 6, . . . ,2007, 2008.
So how many multiples 5s are there between 1 and 2008, well
As m is an integer then there are 401.
Now some terms in the prime factorization will have 25 as a factor, and hence that terms will have 2 factors of 5. So we must count how many terms are multiples of 25. Now we already have counted 1 of those factors of 5 (out of those numbers that are multiples of 5^2), and so when we determine how many factors are multiples of 5^2, they will add 1 extra multiple of 5 into our count.
As n is an integer then there are 80.
But some of the factors of 2008! will be multiples of 5^3 = 125, so when we count these by the same logic as before they will contribute an extra 1 multiple of 5 into our count, so
As p is an integer then there are 16.
But some of the factors of 2008! will be multiples of 5^4 = 625, and so these will add an extra 1 multiple of 5 into our count. So,
As j is an integer there are 3 of these.
As 5^5 is greater than 2008, then there are no multiples of 5^5 up to 2008.
So in total the amount of factors of 5 in 2008! are
Therefore there are 500 factors of 10 in 2008! and hence 2008! ends in 500 zeros.
Good luck everyone for the competition today =)
Australian Maths Competition (1 Viewer)
- Thread starter maths94
- Start date
dog on heat
check it out uh uh uh uh
i hear answeres to maths comp was leaked
Kajixtatsu
New Member
- Joined
- Dec 16, 2006
- Messages
- 13
- Gender
- Male
- HSC
- 2010
Re: How did people go in the maths comp today?
That was unusual... because they had to be integers you were obviously including the squares... 0, 1, 4, 9, 16... 49 and four quadrants but that gives 32 , I don't know if I doubled up =| but I went with B) 30What'd you get for question 14?
gurmies
Drover
Re: Australian Mathematics Competition
I got 48
Keep in mind a pair like 3, root[41] satisfied it
I got 48
Keep in mind a pair like 3, root[41] satisfied it
Last edited:
kurt.physics
Member
- Joined
- Jun 16, 2007
- Messages
- 840
- Gender
- Undisclosed
- HSC
- N/A
Re: How did u go in the Australian Maths Competition?
 at each of five houses. To get to the first house he crosses a magic river once, and to get to any house from another, he also crosses a magic river once. Each time he crosses a magic river, the number of rabbits he has doubles. He has no rabbits left when he leaves the fifth house. What is the minimum number of rabbits he could have.$)


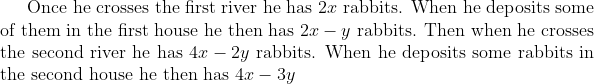

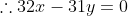




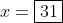

wat did u get for the magic rabbit ones?
Kajixtatsu
New Member
- Joined
- Dec 16, 2006
- Messages
- 13
- Gender
- Male
- HSC
- 2010
Re: Australian Mathematics Competition
I see, thanks well then I shouldve just gone higher than 32 >< [fail]I got 48
Keep in mind a pair like 3, root[41] satisfied it
K
khorne
Guest
Re: Australian Mathematics Competition
I don't think this counts for a valid solution...But for the ascending question, I just divided the 4 digit number given by 6...and it worked...6 marks in 1 minute.
Personally, I don't care about the mark...I only take the test to steal the question booklet afterward =]
I don't think this counts for a valid solution...But for the ascending question, I just divided the 4 digit number given by 6...and it worked...6 marks in 1 minute.
Personally, I don't care about the mark...I only take the test to steal the question booklet afterward =]
Last edited by a moderator:
gurmies
Drover
Re: Australian Mathematics Competition
loli don't think this counts for a valid solution...but for the ascending question, i just divided the 4 digit number given by 6...and it worked...6 marks in 1 minute.
Official
Bring it on
Re: Australian Mathematics Competition
I got 60.
I got 60.
Official
Bring it on
Re: Australian Mathematics Competition
Kurt already answered the rabbit one.hey i did the intermidate paper ...wat did every1 get for the rabbit one? also wat did they get for q30 the train one...also q29 the pattern wat was there answer for that..
kurt.physics
Member
- Joined
- Jun 16, 2007
- Messages
- 840
- Gender
- Undisclosed
- HSC
- N/A
Re: Australian Mathematics Competition
So we have to find the 11th term, which is the sum of the first 11 terms of the arithmetic sequence with a = 6, d = 3.
)
)
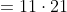
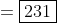
The pattern was 6, 6 + 9, 6 + 9 + 12, 6 + 9 + 12 + 15, . . .hey i did the intermidate paper ...wat did every1 get for the rabbit one? also wat did they get for q30 the train one...also q29 the pattern wat was there answer for that..
So we have to find the 11th term, which is the sum of the first 11 terms of the arithmetic sequence with a = 6, d = 3.
kurt.physics
Member
- Joined
- Jun 16, 2007
- Messages
- 840
- Gender
- Undisclosed
- HSC
- N/A
Re: Australian Mathematics Competition
How did you think you went? =)I don't think this counts for a valid solution...But for the ascending question, I just divided the 4 digit number given by 6...and it worked...6 marks in 1 minute.
Personally, I don't care about the mark...I only take the test to steal the question booklet afterward =]
Aquawhite
Retiring
Re: Australian Mathematics Competition
This!I don't think this counts for a valid solution...But for the ascending question, I just divided the 4 digit number given by 6...and it worked...6 marks in 1 minute.
Personally, I don't care about the mark...I only take the test to steal the question booklet afterward =]
occer
Member
- Joined
- Jun 8, 2009
- Messages
- 499
- Gender
- Undisclosed
- HSC
- N/A
Re: 2009 AMT Mathematics Competition
Hopefully credit.
Join the club.I pretty much gave up from 16 onwards... didnt even try the last 5 questions. I know, really bad.
Hopefully credit.
Re: Australian Mathematics Competition
i reckon the maths comp committee purposely did that just to tease us.
OMGGG why didnt i think of that????!!!!I don't think this counts for a valid solution...But for the ascending question, I just divided the 4 digit number given by 6...and it worked...6 marks in 1 minute.
i reckon the maths comp committee purposely did that just to tease us.
K
khorne
Guest
Re: Australian Mathematics Competition
I got most...except the train question...How did you think you went? =)
Official
Bring it on
Re: Australian Mathematics Competition

Heh, yeh I bet you they did lol. Darn if I found out about that then it would have saved me the 5minutes it took me to answer the questionOMGGG why didnt i think of that????!!!!
i reckon the maths comp committee purposely did that just to tease us.
Re: Australian Mathematics Competition
= =MAN i feel inferior to you guys lol.
how do you actually do that question?Heh, yeh I bet you they did lol. Darn if I found out about that then it would have saved me the 5minutes it took me to answer the question
= =MAN i feel inferior to you guys lol.
