Carrotsticks
Retired
- Joined
- Jun 29, 2009
- Messages
- 9,467
- Gender
- Undisclosed
- HSC
- N/A
In case people haven't noticed, many Question 8's or Question 7's are either one of two things.
1. Elaborate and complicated proofs for very simple expresssions.
2. Proofs for the convergence of a particular series, and finding it's closed form.
For example...
2010 HSC
All of Question 8 was to prove that the Riemann Zeta function for s=2 converges to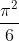 . Also known as the Basel Problem.
. Also known as the Basel Problem.
2009 HSC
Question 8 (b) and (c) was a proof utilising the Squeeze Law to prove that}=\frac{1}{e})
Question 8 (a) was a proof for the convergence for a series (no latex, maybe later)
2008 HSC
Question 8 (b) was a proof for the fact that the surface area of a sphere is 4*pi*r^2
Question 6 (c) was a proof for the convergence for a series.
2007 HSC
Question 8 (c) was a proof for the fact that the limiting ratio between the perimeter and the average of the diagonals... is pi^2/2.
2006 HSC
Question 7 (c) was a proof for the limiting term of a sequence.
Question 8 (b) was a proof that the points of inflexion for the curve x^n*e^(-x) converge to share the same y value as n grows larger.
2005 HSC
Question 6 (a) was a proof for the series expansion for e.
2004 HSC
Question 8 (b) was a proof that a particular integral converges to 0 for large powers n.
2003 HSC
Question 8 (a) was a proof for a closed form of an open expression.
Question 8 (b) was a proof for the irrationality of pi by contradiction, which is reached by taking a limit.
2002 HSC
Question 8 (a) was the first half of the proof for the Riemann Zeta function for s=2. (Note: This was finally completed in 2010 HSC)
2001 HSC
Question 7 (a) was a proof for the closed form of an expression.
2000 HSC
Question 8 (a) was a proof for the closed form of an expression.
Hopefully you guys get the point. I may add in a bit of LaTeX later
The 2011 HSC was the only year not to have some sort proof for a limiting case. Although it is the most recent one (hence the best reflection of future HSC exams), I believe that students should still practise these questions to develop the skills necessary to confidently answer Question 8-type questions.
What I was hoping for, was to make a thread full of 'Question 8' type questions that students can practise on constantly, in preparation for school exams or even for the HSC.
These questions can be elaborate but elementary proofs for simple expressions that we take for granted such as:
- Area of a circle = pi*r^2
- Perimeter of a circle = 2*pi*r
- Volume of a sphere = 4/3 * pi*r^3
- Alternating Harmonic Series = ln(2)
- Leibniz's formula = pi/4 (EDIT: A preliminary approximation was deduced for this in 1997 HSC Q6 (a))
- Series expansions for e and pi (and perhaps other constants such as Euler's gamma constant)
- Riemann sum proofs for integrals
If you have any ideas, feel free to contribute.
Yes, I type these up myself, and yes I do use MathType for them.
Proof for convergence of Riemann Zeta function for s=2: http://www.mediafire.com/?1w857kfwotsog1d
Proof for that the area of a circle is pi*r^2: http://www.mediafire.com/?l2i4xoce2msjgpo
EDIT: I forgot that they are changing the format of the HSC. Consequently, the title should be Question 6. I'm guessing that the difficulty would be about the same.
1. Elaborate and complicated proofs for very simple expresssions.
2. Proofs for the convergence of a particular series, and finding it's closed form.
For example...
2010 HSC
All of Question 8 was to prove that the Riemann Zeta function for s=2 converges to
2009 HSC
Question 8 (b) and (c) was a proof utilising the Squeeze Law to prove that
Question 8 (a) was a proof for the convergence for a series (no latex, maybe later)
2008 HSC
Question 8 (b) was a proof for the fact that the surface area of a sphere is 4*pi*r^2
Question 6 (c) was a proof for the convergence for a series.
2007 HSC
Question 8 (c) was a proof for the fact that the limiting ratio between the perimeter and the average of the diagonals... is pi^2/2.
2006 HSC
Question 7 (c) was a proof for the limiting term of a sequence.
Question 8 (b) was a proof that the points of inflexion for the curve x^n*e^(-x) converge to share the same y value as n grows larger.
2005 HSC
Question 6 (a) was a proof for the series expansion for e.
2004 HSC
Question 8 (b) was a proof that a particular integral converges to 0 for large powers n.
2003 HSC
Question 8 (a) was a proof for a closed form of an open expression.
Question 8 (b) was a proof for the irrationality of pi by contradiction, which is reached by taking a limit.
2002 HSC
Question 8 (a) was the first half of the proof for the Riemann Zeta function for s=2. (Note: This was finally completed in 2010 HSC)
2001 HSC
Question 7 (a) was a proof for the closed form of an expression.
2000 HSC
Question 8 (a) was a proof for the closed form of an expression.
Hopefully you guys get the point. I may add in a bit of LaTeX later
The 2011 HSC was the only year not to have some sort proof for a limiting case. Although it is the most recent one (hence the best reflection of future HSC exams), I believe that students should still practise these questions to develop the skills necessary to confidently answer Question 8-type questions.
What I was hoping for, was to make a thread full of 'Question 8' type questions that students can practise on constantly, in preparation for school exams or even for the HSC.
These questions can be elaborate but elementary proofs for simple expressions that we take for granted such as:
- Area of a circle = pi*r^2
- Perimeter of a circle = 2*pi*r
- Volume of a sphere = 4/3 * pi*r^3
- Alternating Harmonic Series = ln(2)
- Leibniz's formula = pi/4 (EDIT: A preliminary approximation was deduced for this in 1997 HSC Q6 (a))
- Series expansions for e and pi (and perhaps other constants such as Euler's gamma constant)
- Riemann sum proofs for integrals
If you have any ideas, feel free to contribute.
Yes, I type these up myself, and yes I do use MathType for them.
Proof for convergence of Riemann Zeta function for s=2: http://www.mediafire.com/?1w857kfwotsog1d
Proof for that the area of a circle is pi*r^2: http://www.mediafire.com/?l2i4xoce2msjgpo
EDIT: I forgot that they are changing the format of the HSC. Consequently, the title should be Question 6. I'm guessing that the difficulty would be about the same.
Last edited:
