kingkong123
Member
- Joined
- Dec 20, 2011
- Messages
- 98
- Gender
- Male
- HSC
- 2012
Hi,
Recently i've been looking at integrating using Euler's formula, i don't know when it will be useful but im trying to get the hang of it..
Ive been trying to do this integral:
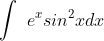
using Euler's formula but i can't get it. I can do it using integration by parts, but was looking for a worked solution using Euler's.
To start i had
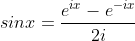 therefore
therefore 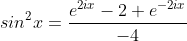
and im presuming you continue on my multiplying through by and integrate....
and integrate....
Can some one please help ))))))))
))))))))
Recently i've been looking at integrating using Euler's formula, i don't know when it will be useful but im trying to get the hang of it..
Ive been trying to do this integral:
using Euler's formula but i can't get it. I can do it using integration by parts, but was looking for a worked solution using Euler's.
To start i had
and im presuming you continue on my multiplying through by
Can some one please help

