HSC 2013 MX2 Marathon (archive) (5 Viewers)

Sy123 · Sep 5, 2013

Re: HSC 2013 4U Marathon

$A number from the sequence$ \ \ F_n = F_{n-1} + F_{n-2} \ , \ F_0 = 0 \ F_1=1 \ \ $is called a Fibonacci number$

$A formula for the$ \ n^{th} \ $Fibonacci number is$

$F_n = \frac{1}{\sqrt{5}} \left(\left(\frac{1+\sqrt{5}}{2} \right )^n - \left( \frac{1-\sqrt{5}}{2} \right)^n \right )$

$Using this formula or otherwise, prove that$ \ x \ $is a Fibonacci number$

$If and only if$ \qquad 5x^2+4 \qquad $or$ \qquad 5x^2-4 \qquad $is a perfect square$

asianese · Sep 5, 2013

Re: HSC 2013 4U Marathon

Think you meant:

prove that x is a Fibonacci number if and only if 5x^2+4 or 5x^2-4 is a perfect square. (just being picky).

Sy123 · Sep 5, 2013

Re: HSC 2013 4U Marathon

asianese said:
Think you meant:

prove that x is a Fibonacci number if and only if 5x^2+4 or 5x^2-4 is a perfect square. (just being picky).

Yeah that sounds more correct, thank you

asianese · Sep 5, 2013

Re: HSC 2013 4U Marathon

Think of the subsets of $\{ 1,2,\dots,n\}$ as picking $0,1,2,\dots$ elements from the set. That is, if you choose 0 elements, you have 1 choice. If you choose 1 element, you have n choices, for 2, nC2. Ie: $\binom{n}{0} + \binom{n}{1}+\cdots+\binom{n}{n} = 2^n$ which is clearer from your point of view from the expansion of $(1+1)^n.$ On replacement of n by 2n we get the result.

Sy123 · Sep 8, 2013

Re: HSC 2013 4U Marathon

$$The complex numberss$ \ \ a_1, a_2, a_3, \dots, a_n$

$$satsify$ \ \ |a_i| \leq A \ \ $for$ \ i=1,2, \dots, n \ \ $for positive real$ \ A \geq 1$

$For the complex equation$

$a_n z^n + a_{n-1}z^{n-1} + \dots + a_1 z = B \ \ $for positive real$ \ B \leq 1$

$Show that there is no solution in$ \ z \ $for$

$|z| \leq \frac{|B|}{|A| + |B|}$

EDIT 1: One sec, I may have made a mistake
EDIT 2: It should be fine now
EDIT 3: Changed some restrictions, I hope its fine now (B < 1, A > 1)

rural juror · Sep 8, 2013

Re: HSC 2013 4U Marathon

RealiseNothing said:
We know that:

$a+b \geq 2\sqrt{ab}$

Now let $a=x^2y^6$ and $b=x^6y^2$

$x^2y^6 + x^6y^2 \geq 2\sqrt{x^8y^8}$

$x^2y^6 + x^6y^2 \geq 2x^4y^4$

Multiply both sides by 3 to establish the inequality:

$3x^2y^6 + 3x^6y^2 \geq 6x^4y^4$

Now consider:

$(x^2-y^2)^4 \geq 0$

$x^8 - 4x^6y^2 + 6x^4y^4 - 4x^2y^6 + y^8 \geq 0$

$x^8 + y^8 + 6x^4y^4 \geq 4x^6y^2 + 4x^2y^6$

Split the RHS up into the following:

$x^8 + y^8 + 6x^4y^4 \geq x^6y^2 + x^2y^6 + 3x^6y^2 + 3x^2y^6$

$x^8 + y^8 + 6x^4y^4 - 3x^6y^2 - 3x^2y^6 \geq x^6y^2 + x^2y^6$

$x^8 + y^8 - (3x^6y^2 + 3x^2y^6 - 6x^4y^4) \geq x^6y^2 + x^2y^6$

Now using the inequality we establish before, we get:

$3x^6y^2 + 3x^2y^6 - 6x^4y^4 \geq 0$

Let this be A:

$x^8 + y^8 - A \geq x^6y^2 + x^6y^2$

Hence since $A \geq 0$

$x^8+y^8 \geq x^6y^2 + x^2y^6$

$x^8+y^8 \geq x^2y^2(x^4 + y^4)$

$\frac{x^8+y^8}{x^2y^2} \geq x^4 + y^4$

$\frac{x^6}{y^2} + \frac{y^6}{x^2} \geq x^4 + y^4$

i might have a quicker way and easier to find,

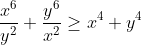
looking at x^6(y^-2 - x^-2) > y^6(y^-2 - x^-2) *just assume the greater than sign is greater than or equal to, this inequality can be easily shown for x>y and y>x to hold true, then all you have to do is move the fractions over and you have the result. max 3 lines or so

rural juror · Sep 8, 2013

Re: HSC 2013 4U Marathon

oh same solution as sys thatll teach me to scroll down

Sy123 · Sep 9, 2013

Re: HSC 2013 4U Marathon

$$Let$ \ \ I_n = \int_0^{\pi /4} \tan^{2n+1} x \ dx$

$Find a recurrence formula for this integral, and use it to prove$

$\ln 2 = \sum_{n=1}^{\infty} \frac{(-1)^{n-1}}{n}$

Sy123 · Sep 10, 2013

Re: HSC 2013 4U Marathon

$Take the set of all positive integers$ \ S \ $which contains all positive integers without a zero in their representation$

$$S = \{1,2, \dots , 9, 11, 12, \dots , 19, 21, \dots \}$

$$Let each element in the set$ \ S \ $be$ \ \ a_k \ , \ k=1,2, \dots$

$Consider the sum$

$S_n = \sum_{k=1}^n \frac{1}{a_k}$

$$Show that as$ \ n \to \infty \ \ S_n \to \infty$

Drongoski · Sep 10, 2013

Re: HSC 2013 4U Marathon

Sy123 said:
$$Let$ \ \ I_n = \int_0^{\pi /4} \tan^{2n+1} x \ dx$

$Find a recurrence formula for this integral, and use it to prove$

$\ln 2 = \sum_{n=1}^{\infty} \frac{(-1)^{n-1}}{n}$

$I_n = \int ^{\frac {\pi}{4}} _0 tan^{2n+1}x dx = \int ^a _a tan ^{2n-1}x(sec^2 x -1)dx \\ \\ = \int ^a _0 tan^{2n-1}x sec^2x dx - \int ^a _0 tan ^{2n-1}xdx \\ \\ = \left [\frac {tan ^{2n} }{2n} \right ] ^a _0- I_{n-1} = \frac {1}{2n} - I_{n-1}\\ \\ I_0 = \int ^a _0 tan x dx = \left [ -ln |cos x| \right ] ^a _0 = ln \sqrt 2 \\ \\ I_n = \frac {1}{2n} - I_{n-1} = \frac {1}{2n} - \frac {1}{2(n-1)} + I_{n-2} = \cdots \\ \\ = \frac {1}{2n} - \frac {1}{2(n-1)} + \frac {1}{2(n-2)} - \cdots + \frac {(-1) ^{n-1}}{2} - I_0\\ \\ = \frac {1}{2} \sum ^n _1 \frac {(-1) ^{(n-1)}}{n} - I_0 \\ \\ $Now $ 0 < I_n \leq \int _0 ^a ( \frac {4x}{\pi}) ^n dx = \left [ (\frac {4}{\pi})^ n \frac {x}{n+1} \right ] ^ {\frac {\pi}{4}} _0 = \frac {\pi}{4(n+1)} \to 0 $ as n $ \to \infty.\\ \\ \therefore $ by the Sandwich Theorem $ \\ \\ \lim _{n \to \infty} I_n = 0 \implies \sum ^\infty _{n=1} \frac {(-1) ^{n-1}}{n} = 2 \times I_0 = 2 \times ln \sqrt 2 = ln 2$

Above solution messy and may contain errors. Have been making it up as I went along.

Sy123 · Sep 11, 2013

Re: HSC 2013 4U Marathon

Drongoski said:
$I_n = \int ^{\frac {\pi}{4}} _0 tan^{2n+1}x dx = \int ^a _a tan ^{2n-1}x(sec^2 x -1)dx \\ \\ = \int ^a _0 tan^{2n-1}x sec^2x dx - \int ^a _0 tan ^{2n-1}xdx \\ \\ = \left [\frac {tan ^{2n} }{2n} \right ] ^a _0- I_{n-1} = \frac {1}{2n} - I_{n-1}\\ \\ I_0 = \int ^a _0 tan x dx = \left [ -ln |cos x| \right ] ^a _0 = ln \sqrt 2 \\ \\ I_n = \frac {1}{2n} - I_{n-1} = \frac {1}{2n} - \frac {1}{2(n-1)} + I_{n-2} = \cdots \\ \\ = \frac {1}{2n} - \frac {1}{2(n-1)} + \frac {1}{2(n-2)} - \cdots + \frac {(-1) ^{n-1}}{2} - I_0\\ \\ = \frac {1}{2} \sum ^n _1 \frac {(-1) ^{(n-1)}}{n} - I_0 \\ \\ $Now $ 0 < I_n \leq \int _0 ^a ( \frac {4x}{\pi}) ^n dx = \left [ (\frac {4}{\pi})^ n \frac {x}{n+1} \right ] ^a _0 = \frac {\pi}{4(n+1)} \to 0\\ \\ \therefore $ by the Sandwich Theorem $ \\ \\ \lim _{n \to \infty} I_n = 0 \implies \sum ^\infty _{n=1} \frac {(-1) ^{n-1}}{n} = 2 \times I_0 = 2 \times ln \sqrt 2 = ln 2$

Maybe not quite correct yet. May need to have a look at it again

Yep that is correct.

$What is the largest integer$ \ n \ $for which$ \ n^3+100 \ $is divisible by$ \ n+10 \ $?$

Qb · Sep 11, 2013

Re: HSC 2013 4U Marathon

Sy123 said:
$What is the largest integer$ \ n \ $for which$ \ n^3+100 \ $is divisible by$ \ n+10 \ $?$

First post, let's see if I can get the latex right -- I'm studying how you've done it lol

$Using polynomial division: $ \ n^3+100 = (n+10)(n^2-10n+100) - 900 \$

$Therefore $ \ \frac{n^3+100}{n+10} = n^2-10n+100 - \frac{900}{n+10} \$

$Both sides have to be integers, so the fraction must also be an integer. Given we're looking for the largest value of $ \ n\ $, this corresponds to the fraction being equal to 1, or $ \ n+10 = 900 \ $ giving $ \ n = 890 \ $.$

Sy123 said:
$Take the set of all positive integers$ \ S \ $which contains all positive integers without a zero in their representation$

$$S = \{1,2, \dots , 9, 11, 12, \dots , 19, 21, \dots \}$

$$Let each element in the set$ \ S \ $be$ \ \ a_k \ , \ k=1,2, \dots$

$Consider the sum$

$S_n = \sum_{k=1}^n \frac{1}{a_k}$

$$Show that as$ \ n \to \infty \ \ S_n \to \infty$

Are you sure this one is correct? I'm fairly certain I can prove it has a lower bound of 9 and an upper bound of 90 pretty easily...

Sy123 · Sep 11, 2013

Re: HSC 2013 4U Marathon

Qb said:
First post, let's see if I can get the latex right -- I'm studying how you've done it lol

$Using polynomial division: $ \ n^3+100 = (n+10)(n^2-10n+100) - 900 \$

$Therefore $ \ \frac{n^3+100}{n+10} = n^2-10n+100 - \frac{900}{n+10} \$

$Both sides have to be integers, so the fraction must also be an integer. Given we're looking for the largest value of $ \ n\ $, this corresponds to the fraction being equal to 1, or $ \ n+10 = 900 \ $ giving $ \ n = 890 \ $.$

Are you sure this one is correct? I'm fairly certain I can prove it has a lower bound of 9 and an upper bound of 90 pretty easily...

The first answer is correct, as for the second one, let me check my proof again, I got the result from 'Art of Problem SOlving' and it asked whether it diverged and converged.

Feel free to post your proof of the upper and lower bounds though

EDIT: Yeah my proof is wrong, I didn't consider the numbers where a zero is in the middle -.-

Sy123 · Sep 11, 2013

Re: HSC 2013 4U Marathon

Another good Putnam question

$Let$ \ k \ $be a positive integer.$

$The$ \ n$-th derivative of$ \ \ \frac{1}{x^k-1} \ \ $has the form$

$\frac{P_n(x)}{(x^k-1)^{n+1}} \ \ $, where$ \ \ P_n(x) \ \ $is a polynomial.$$

$$Find$ \ \ P_n(1)$

rural juror · Sep 13, 2013

Re: HSC 2013 4U Marathon

Sy123 said:
Another good Putnam question

$Let$ \ k \ $be a positive integer.$

$The$ \ n$-th derivative of$ \ \ \frac{1}{x^k-1} \ \ $has the form$

$\frac{P_n(x)}{(x^k-1)^{n+1}} \ \ $, where$ \ \ P_n(x) \ \ $is a polynomial.$$

$$Find$ \ \ P_n(1)$

Is it (-1)^n-1*n!*k^n???

fionarykim · Sep 13, 2013

Re: HSC 2013 4U Marathon

How are u guys typing so much maths here LOOOL im amazed

Also the questions just seem to just confuse my head, too hard ):

Sy123 · Sep 13, 2013

Re: HSC 2013 4U Marathon

rural juror said:
Is it (-1)^n-1*n!*k^n???

Yep =)

rural juror · Sep 13, 2013

Re: HSC 2013 4U Marathon

seemed a bit on the easy side for putnam surely???

Sy123 · Sep 13, 2013

Re: HSC 2013 4U Marathon

rural juror said:
seemed a bit on the easy side for putnam surely???

I thought so too, its problem A1 for 2002 iirc.

HeroicPandas · Sep 14, 2013

Re: HSC 2013 4U Marathon

$$Let a, b, c be positive real numbers with sum 3. \\ \\ Prove that \sqrt{a} + \sqrt{b}+\sqrt{c} \geq ab + bc + ca$

HSC 2013 MX2 Marathon (archive) (5 Viewers)

This too shall pass

Σ

This too shall pass

Σ

This too shall pass

New Member

New Member

This too shall pass

This too shall pass

Well-Known Member

This too shall pass

New Member

This too shall pass

This too shall pass

New Member

Member

This too shall pass

New Member

This too shall pass

Heroic!

Users Who Are Viewing This Thread (Users: 0, Guests: 5)