-
Looking for HSC notes and resources? Check out our Notes & Resources page
complex number euler form modulus question (1 Viewer)
- Thread starter gamja
- Start date
yanujw
Well-Known Member
- Joined
- May 23, 2020
- Messages
- 339
- Gender
- Male
- HSC
- 2022
The most prominent method is to let 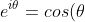 + isin(\theta)) . Then use the fact that
. Then use the fact that 
The locus of z itself is an ellipse which would have been helpful in the old syllabus. However, there is still a geometric way to find the maximum value.
Recall that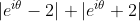 means the combined distance of
means the combined distance of 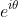 from the points 2 and -2 on the Argand diagram. And
from the points 2 and -2 on the Argand diagram. And 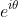 represents the unit circle of points. The combined length of the two vectors as visualised below is maximised* at either the points i or -i (see diagram below). At these points, each line has a length of
represents the unit circle of points. The combined length of the two vectors as visualised below is maximised* at either the points i or -i (see diagram below). At these points, each line has a length of 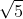 so the maximum combined length is
so the maximum combined length is 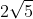
*this is just a vague conclusion based on looking at the graph, it may require an actual proof which just goes back to the algebraic version anyways.

The locus of z itself is an ellipse which would have been helpful in the old syllabus. However, there is still a geometric way to find the maximum value.
Recall that
*this is just a vague conclusion based on looking at the graph, it may require an actual proof which just goes back to the algebraic version anyways.

Last edited:
The geometric method (see your diagram) is the easiest to understand and the shortest.The most prominent method is to let. Then use the fact that
The locus of z itself is an ellipse which would have been helpful in the old syllabus. However, there is still a geometric way to find the maximum value.
Recall thatmeans the combined distance of
from the points 2 and -2 on the Argand diagram. And
represents the unit circle of points. The combined length of the two vectors as visualised below is maximised* at either the points i or -i (see diagram below). At these points, each line has a length of
so the maximum combined length is
*this is just a vague conclusion based on looking at the graph, it may require an actual proof which just goes back to the algebraic version anyways.
View attachment 37914
Some dude (can't remember who) came here before and tried to use the AM-GM inequality to do it but it didn't work. They kind of had a good idea but had the inequality the wrong way around.
However the AM-QM inequality does work (instead of the AM-GM one):
^2+\sin^2\theta+(\cos\theta+2)^2+\sin^2\theta}{2}}\\&=2\sqrt{\frac{\cos^2\theta+\sin^2\theta-4\cos\theta+4+\cos^2\theta+\sin^2\theta+4\cos\theta+4}{2}}\\&=2\sqrt{\frac{10}{2}}\\&=2\sqrt5\text{ with equality }\iff|e^{i\theta}-2|=|e^{i\theta}+2|\iff\cos\theta=0\iff\theta=\frac{(2k+1)\pi}{2}\forall k\in\Bbb Z\end{aligned})
However the AM-QM inequality does work (instead of the AM-GM one):
Last edited:
member 6003
Member
- Joined
- Apr 20, 2022
- Messages
- 83
- Gender
- Male
- HSC
- 2023
I was the dude, cool solution but ofc its outside the syllabus. I saw 2sqrt(5) in my solution so I just thought it would be correct without considering any logic before posting.Some dude (can't remember who) came here before and tried to use the AM-GM inequality to do it but it didn't work. They kind of had a good idea but had the inequality the wrong way around.
However the AM-QM inequality does work (instead of the AM-GM one):
^2+\sin^2\theta+(\cos\theta+2)^2+\sin^2\theta}{2}}\\&=2\sqrt{\frac{\cos^2\theta+\sin^2\theta-4\cos\theta+4+\cos^2\theta+\sin^2\theta+4\cos\theta+4}{2}}\\&=2\sqrt{\frac{10}{2}}\\&=2\sqrt5\text{ with equality }\iff|e^{i\theta}-2|=|e^{i\theta}+2|\iff\cos\theta=0\iff\theta=\frac{(2k+1)\pi}{2}\forall k\in\Bbb Z\end{aligned})
member 6003
Member
- Joined
- Apr 20, 2022
- Messages
- 83
- Gender
- Male
- HSC
- 2023
well if you prove first then yea they couldn't mark you down for it