Australian Maths Competition (2 Viewers)

[jathu123]

Re: Australian Maths Competition 2013

No, I am sure it's 126. Unless I miscounted. Ceebs.

ye it is probably 126. Nevertheless this is what I did, probably I missed something haha

http://imgur.com/a/fsfVN

 

[Paradoxica]

Re: Australian Maths Competition 2013

ye it is probably 126. Nevertheless this is what I did, probably I missed something haha

http://imgur.com/a/fsfVN

Chords can intersect, but each one can only intersect with exactly one other chord. Pretty sure vertex contact does not count as intersection.

Also did you just assume the formula is true or carry a bit of inductive reasoning to demonstrate it?

 

[jathu123]

Re: Australian Maths Competition 2013

Chords can intersect, but each one can only intersect with exactly one other chord. Pretty sure vertex contact does not count as intersection.

Also did you just assume the formula is true or carry a bit of inductive reasoning to demonstrate it?

yeah I don't think I fully got the question, and yeah I just assumed it haha, I didn't fully go into it, was just thinking of a quick answer by looking at simpler cases

 

[Paradoxica]

Re: Australian Maths Competition 2013

yeah I don't think I fully got the question, and yeah I just assumed it haha, I didn't fully go into it, was just thinking of a quick answer by looking at simpler cases

I was generating a few cases and immediately deduced that you have to dissect the polygon into as many quadrilaterals as possible, then draw diagonals in each one, to maximise it.

Of course, I did not formally prove this statement, but meh.

 

[SimpletonPrime]

Re: Australian Maths Competition 2013

I think Q30 is an old question. I remembered this one from an AMO contest, may be. Although, I didn't get it during the test, I was out of time.

Do you guys have answer for question 27,28,29 in senior division?

 

[Paradoxica]

Re: Australian Maths Competition 2013

I think Q30 is an old question. I remembered this one from an AMO contest, may be. Although, I didn't get it during the test, I was out of time.

Do you guys have answer for question 27,28,29 in senior division?

The prime factorisation of 2016 is 32*63

Knowing this, and the fact that f(61) = 63, we obtain the following series of function values:

f(63) = f(f(61)) = 122
f(122) = f(f(63)) = 126
f(126) = f(f(122)) = 244
f(244) = f(f(126)) = 252
f(252) = f(f(244)) = 488
f(488) = f(f(252)) = 504
f(504) = f(f(488)) = 976
f(976) = f(f(504)) = 1008
f(1008) = f(f(976)) = 1952
f(1952) = f(f(1008)) = 2016
f(2016) = f(f(1952)) = 3904

 

[Paradoxica]

Re: Australian Maths Competition 2013

I think Q30 is an old question. I remembered this one from an AMO contest, may be. Although, I didn't get it during the test, I was out of time.

Do you guys have answer for question 27,28,29 in senior division?

I don't remember any other questions. If you would kindly... provide them.

 

[SimpletonPrime]

Re: Australian Maths Competition 2013

The prime factorisation of 2016 is 32*63

Knowing this, and the fact that f(61) = 63, we obtain the following series of function values:

f(63) = f(f(61)) = 122
f(122) = f(f(63)) = 126
f(126) = f(f(122)) = 244
f(244) = f(f(126)) = 252
f(252) = f(f(244)) = 488
f(488) = f(f(252)) = 504
f(504) = f(f(488)) = 976
f(976) = f(f(504)) = 1008
f(1008) = f(f(976)) = 1952
f(1952) = f(f(1008)) = 2016
f(2016) = f(f(1952)) = 3904

I was up to f(126)=252 and then time-out.

After the test, I realise that we can figure out the pattern (or prove by induction) that and

 

[SimpletonPrime]

Re: Australian Maths Competition 2013

OK. I remembered two questions:

Given positive integer and where . How many values of ?

How many pairs (a,b) of positive integers such that and .

 

[Paradoxica]

Re: Australian Maths Competition 2013

OK. I remembered two questions:

Given positive integer and where . How many values of ?

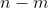
How many pairs (a,b) of positive integers such that and .

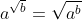
Well the first one is fairly simple in terms of the first step.

(a(n+m)+b)(n-m) = 2016² = 3⁴×7²×2¹⁰

n-m must be a perfect square so that means we should pull out all the possible square factors from the factorisation of 2016²

There's also the special case where the factors are equal.

If I'm reading that correctly, I'm seeing that m must also be less than all the integer coefficients of the quadratic, which is going to be a huge road block for people who like to bash problems.

The second one requires cases

a^√b = a^b/2

There are two ways this equation can be true.

1. The exponents are equal, so √b = b/2
=> b=4, a can be anything in the valid range.
2. a=1, therefore b can be anything in the valid range.

Observe the case a=1, b=4 is double counted, so subtract one from the final amount of solutions.

I think that's all, though I may be missing something glaringly obvious.

 

[SimpletonPrime]

Re: Australian Maths Competition 2013

Well the first one is fairly simple in terms of the first step.

(a(n+m)+b)(n-m) = 2016² = 3⁴×7²×2¹⁰

n-m must be a perfect square so that means we should pull out all the possible square factors from the factorisation

Why do you imply n-m must be a perfect square?

Oh, in the test, m only less than n.

 

[Paradoxica]

Re: Australian Maths Competition 2013

Why do you imply n-m must be a perfect square?

Actually, m only less than n.

True, that's not necessarily the case.
but I must ask again, are a,b,c allowed to be negative? If not, then the problem jumps a few notches in complexity...

 

[SimpletonPrime]

Re: Australian Maths Competition 2013

True, that's not necessarily the case.
but I must ask again, are a,b,c allowed to be negative? If not, then the problem jumps a few notches in complexity...

No, a,b,c are positive integers.

I got 199 for the question , almost forgot the case a=1...

 

[Paradoxica]

Re: Australian Maths Competition 2013

Why do you imply n-m must be a perfect square?

Oh, in the test, m only less than n.

I already took that back...

Since a,b,c can be assumed to be any integers, then all we have to do is partition the factors of 2016² into two sides and use multiplicity arguments to remove repeated solutions from the set, and then double the answer because of negative factorisations.

 

[Paradoxica]

Re: Australian Maths Competition 2013

No, a,b,c are positive integers.

I got 199 for the question , almost forgot the case a=1...

... ok then that just ruined my day a bit, looks like we need a bit more cleverness to determine the possible values of n-m...

 

[fluffchuck]

Re: Australian Maths Competition 2013

No, a,b,c are positive integers.

I got 199 for the question , almost forgot the case a=1...

O: I got 199 too, is that the correct answer?

 

[SimpletonPrime]

Re: Australian Maths Competition 2013

O: I got 199 too, is that the correct answer?

I hope so ...

 

[RealiseNothing]

Re: Australian Maths Competition 2013

If its asking for the maximum number of chords without intersection, not number of ways, then I think its 125, idk though, 75% sure its wrong.

Yeah I got this.

 

[seanieg89]

Re: Australian Maths Competition 2013

OK. I remembered two questions:

Given positive integer and where . How many values of ?

Uh, if a,b,c,m are positive integers, how can we possibly have am^2+bm+c=f(m)=0?

 

[SimpletonPrime]

Re: Australian Maths Competition 2013

Uh, if a,b,c,m are positive integers, how can we possibly have am^2+bm+c=f(m)=0?

I think a,b,c are integers. I'm sorry, I don't really remember the problem.