-
Looking for HSC notes and resources? Check out our Notes & Resources page
Make a Difference – Donate to Bored of Studies!
Students helping students, join us in improving Bored of Studies by donating and supporting future students!
HSC 2012-14 MX2 Integration Marathon (archive) (1 Viewer)
- Thread starter bleakarcher
- Start date
- Status
- Not open for further replies.
barbernator
Active Member
- Joined
- Sep 13, 2010
- Messages
- 1,435
- Gender
- Male
- HSC
- 2012
Re: MX2 Integration Marathon
u got the correct answer with the wrong working.
TheProfessional
Member
- Joined
- Sep 3, 2012
- Messages
- 37
- Gender
- Male
- HSC
- 2013
Re: MX2 Integration Marathon
will fix it.
I had a plus instead of times lolu got the correct answer with the wrong working.
will fix it.
barbernator
Active Member
- Joined
- Sep 13, 2010
- Messages
- 1,435
- Gender
- Male
- HSC
- 2012
Re: MX2 Integration Marathon
<a href="http://www.codecogs.com/eqnedit.php?latex=a)~within~the~domain~0\leq x\leq \frac{\pi }{2},~~ 0\leq sinx\leq 1\\ Taking~sinx\leq 1,~sin^{n@plus;1}x\leq sin^{n}x\\ \therefore \int_{0}^{\frac{\pi }{2}}sin^{n@plus;1}x\leq \int_{0}^{\frac{\pi }{2}}sin^{n}x\\ hence,~it~follows~that~ \int_{0}^{\frac{\pi }{2}}sin^{n@plus;2}x\leq \int_{0}^{\frac{\pi }{2}}sin^{n@plus;1}x\leq \int_{0}^{\frac{\pi }{2}}sin^{n}x~as~required" target="_blank"><img src="http://latex.codecogs.com/gif.latex?a)~within~the~domain~0\leq x\leq \frac{\pi }{2},~~ 0\leq sinx\leq 1\\ Taking~sinx\leq 1,~sin^{n+1}x\leq sin^{n}x\\ \therefore \int_{0}^{\frac{\pi }{2}}sin^{n+1}x\leq \int_{0}^{\frac{\pi }{2}}sin^{n}x\\ hence,~it~follows~that~ \int_{0}^{\frac{\pi }{2}}sin^{n+2}x\leq \int_{0}^{\frac{\pi }{2}}sin^{n+1}x\leq \int_{0}^{\frac{\pi }{2}}sin^{n}x~as~required" title="a)~within~the~domain~0\leq x\leq \frac{\pi }{2},~~ 0\leq sinx\leq 1\\ Taking~sinx\leq 1,~sin^{n+1}x\leq sin^{n}x\\ \therefore \int_{0}^{\frac{\pi }{2}}sin^{n+1}x\leq \int_{0}^{\frac{\pi }{2}}sin^{n}x\\ hence,~it~follows~that~ \int_{0}^{\frac{\pi }{2}}sin^{n+2}x\leq \int_{0}^{\frac{\pi }{2}}sin^{n+1}x\leq \int_{0}^{\frac{\pi }{2}}sin^{n}x~as~required" /></a>
<a href="http://www.codecogs.com/eqnedit.php?latex=b)~let~v=sin^{n-1}x~~~u=-cosx\\\\ dv=(n-1)cosxsin^{n-2}xdx~~~du=sinx\\\\ I_{n}=-cosxsin^{n-1}x]^\frac{\pi }{2}_{0}@plus;(n-1)\int_{\frac{\pi }{2}}^{0}cos^{2}xsin^{n-2}xdx\\\\ I_{n}=(n-1)\int_{\frac{\pi }{2}}^{0}(1-sin^{2}x)sin^{n-2}xdx\\\\ I_{n}=(n-1)I_{n-2}-(n-1)I_{n}\\\\ nI_{n}=(n-1)I_{n-2}~whoops~it~asked~for~n@plus;2~:/\\\\ I_{n}=\frac{n-1}{n}I_{n-2}\\\\ let~n=k@plus;2\\\\ I_{n@plus;2}=\frac{n@plus;1}{n@plus;2}I_{n}~QED" target="_blank"><img src="http://latex.codecogs.com/gif.latex?b)~let~v=sin^{n-1}x~~~u=-cosx\\\\ dv=(n-1)cosxsin^{n-2}xdx~~~du=sinx\\\\ I_{n}=-cosxsin^{n-1}x]^\frac{\pi }{2}_{0}+(n-1)\int_{\frac{\pi }{2}}^{0}cos^{2}xsin^{n-2}xdx\\\\ I_{n}=(n-1)\int_{\frac{\pi }{2}}^{0}(1-sin^{2}x)sin^{n-2}xdx\\\\ I_{n}=(n-1)I_{n-2}-(n-1)I_{n}\\\\ nI_{n}=(n-1)I_{n-2}~whoops~it~asked~for~n+2~:/\\\\ I_{n}=\frac{n-1}{n}I_{n-2}\\\\ let~n=k+2\\\\ I_{n+2}=\frac{n+1}{n+2}I_{n}~QED" title="b)~let~v=sin^{n-1}x~~~u=-cosx\\\\ dv=(n-1)cosxsin^{n-2}xdx~~~du=sinx\\\\ I_{n}=-cosxsin^{n-1}x]^\frac{\pi }{2}_{0}+(n-1)\int_{\frac{\pi }{2}}^{0}cos^{2}xsin^{n-2}xdx\\\\ I_{n}=(n-1)\int_{\frac{\pi }{2}}^{0}(1-sin^{2}x)sin^{n-2}xdx\\\\ I_{n}=(n-1)I_{n-2}-(n-1)I_{n}\\\\ nI_{n}=(n-1)I_{n-2}~whoops~it~asked~for~n+2~:/\\\\ I_{n}=\frac{n-1}{n}I_{n-2}\\\\ let~n=k+2\\\\ I_{n+2}=\frac{n+1}{n+2}I_{n}~QED" /></a>
<a href="http://www.codecogs.com/eqnedit.php?latex=a)~within~the~domain~0\leq x\leq \frac{\pi }{2},~~ 0\leq sinx\leq 1\\ Taking~sinx\leq 1,~sin^{n@plus;1}x\leq sin^{n}x\\ \therefore \int_{0}^{\frac{\pi }{2}}sin^{n@plus;1}x\leq \int_{0}^{\frac{\pi }{2}}sin^{n}x\\ hence,~it~follows~that~ \int_{0}^{\frac{\pi }{2}}sin^{n@plus;2}x\leq \int_{0}^{\frac{\pi }{2}}sin^{n@plus;1}x\leq \int_{0}^{\frac{\pi }{2}}sin^{n}x~as~required" target="_blank"><img src="http://latex.codecogs.com/gif.latex?a)~within~the~domain~0\leq x\leq \frac{\pi }{2},~~ 0\leq sinx\leq 1\\ Taking~sinx\leq 1,~sin^{n+1}x\leq sin^{n}x\\ \therefore \int_{0}^{\frac{\pi }{2}}sin^{n+1}x\leq \int_{0}^{\frac{\pi }{2}}sin^{n}x\\ hence,~it~follows~that~ \int_{0}^{\frac{\pi }{2}}sin^{n+2}x\leq \int_{0}^{\frac{\pi }{2}}sin^{n+1}x\leq \int_{0}^{\frac{\pi }{2}}sin^{n}x~as~required" title="a)~within~the~domain~0\leq x\leq \frac{\pi }{2},~~ 0\leq sinx\leq 1\\ Taking~sinx\leq 1,~sin^{n+1}x\leq sin^{n}x\\ \therefore \int_{0}^{\frac{\pi }{2}}sin^{n+1}x\leq \int_{0}^{\frac{\pi }{2}}sin^{n}x\\ hence,~it~follows~that~ \int_{0}^{\frac{\pi }{2}}sin^{n+2}x\leq \int_{0}^{\frac{\pi }{2}}sin^{n+1}x\leq \int_{0}^{\frac{\pi }{2}}sin^{n}x~as~required" /></a>
<a href="http://www.codecogs.com/eqnedit.php?latex=b)~let~v=sin^{n-1}x~~~u=-cosx\\\\ dv=(n-1)cosxsin^{n-2}xdx~~~du=sinx\\\\ I_{n}=-cosxsin^{n-1}x]^\frac{\pi }{2}_{0}@plus;(n-1)\int_{\frac{\pi }{2}}^{0}cos^{2}xsin^{n-2}xdx\\\\ I_{n}=(n-1)\int_{\frac{\pi }{2}}^{0}(1-sin^{2}x)sin^{n-2}xdx\\\\ I_{n}=(n-1)I_{n-2}-(n-1)I_{n}\\\\ nI_{n}=(n-1)I_{n-2}~whoops~it~asked~for~n@plus;2~:/\\\\ I_{n}=\frac{n-1}{n}I_{n-2}\\\\ let~n=k@plus;2\\\\ I_{n@plus;2}=\frac{n@plus;1}{n@plus;2}I_{n}~QED" target="_blank"><img src="http://latex.codecogs.com/gif.latex?b)~let~v=sin^{n-1}x~~~u=-cosx\\\\ dv=(n-1)cosxsin^{n-2}xdx~~~du=sinx\\\\ I_{n}=-cosxsin^{n-1}x]^\frac{\pi }{2}_{0}+(n-1)\int_{\frac{\pi }{2}}^{0}cos^{2}xsin^{n-2}xdx\\\\ I_{n}=(n-1)\int_{\frac{\pi }{2}}^{0}(1-sin^{2}x)sin^{n-2}xdx\\\\ I_{n}=(n-1)I_{n-2}-(n-1)I_{n}\\\\ nI_{n}=(n-1)I_{n-2}~whoops~it~asked~for~n+2~:/\\\\ I_{n}=\frac{n-1}{n}I_{n-2}\\\\ let~n=k+2\\\\ I_{n+2}=\frac{n+1}{n+2}I_{n}~QED" title="b)~let~v=sin^{n-1}x~~~u=-cosx\\\\ dv=(n-1)cosxsin^{n-2}xdx~~~du=sinx\\\\ I_{n}=-cosxsin^{n-1}x]^\frac{\pi }{2}_{0}+(n-1)\int_{\frac{\pi }{2}}^{0}cos^{2}xsin^{n-2}xdx\\\\ I_{n}=(n-1)\int_{\frac{\pi }{2}}^{0}(1-sin^{2}x)sin^{n-2}xdx\\\\ I_{n}=(n-1)I_{n-2}-(n-1)I_{n}\\\\ nI_{n}=(n-1)I_{n-2}~whoops~it~asked~for~n+2~:/\\\\ I_{n}=\frac{n-1}{n}I_{n-2}\\\\ let~n=k+2\\\\ I_{n+2}=\frac{n+1}{n+2}I_{n}~QED" /></a>
Last edited:
nightweaver066
Well-Known Member
- Joined
- Jul 7, 2010
- Messages
- 1,585
- Gender
- Male
- HSC
- 2012
Re: MX2 Integration Marathon

)
sin^{n - 2}cos^2xdx)
 \int^{\pi /2}_0 sin^{n - 2}(1 - sin^2x}dx)
 \int ^{\pi /2}_0 sin^{n-2}xdx - (n - 1)\int^{\pi /2}_0 sin^nxdx)
I_{n - 2} - (n - 1)I_n)
I_{n - 2})

 with (n + 2)$)






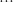
 \times (2n - 3) \times (2n - 5) \times ... \times 3 \times 1}{(2n) \times (2n - 2) \times (2n - 4) \times ... \times 2} \times I_0)



 \times (2n - 3) \times (2n - 5) \times ... \times 3 \times 1}{(2n) \times (2n - 2) \times (2n - 4) \times ... \times 2 \times 1} \times \frac{\pi}{2})
Last edited:
Re: MX2 Integration Marathon

Lol, wallis product... niceIn that case, something more hardcore
\quad Prove by mathematical induction that for integers $n\geq0$\\\\ $I_{2n+1}=\dfrac{2n\times(2n-2)\times...\times4\times2}{(2n+1)\times(2n-1)\times...\times5\times3\times1}\\\\$(f)\quad One application of this reduction formula is by relating it to a well known result called Stirling's formula which approximates $n!$. Suppose that for some positive constant $R\\\\ n!=R\sqrt{n}\left(\dfrac{n}{e}\right)^n\\\\$Using the results of (c), (d) and (e), show that as $n\rightarrow\infty$ then $R\rightarrow\sqrt{2\pi})
Re: MX2 Integration Marathon

Yeah, I went in a circle but ended up with a different result because I equated sin^2 x - 1 to 1. Making so many mistakes tonightI'm not sure about this bit
Let A = sinx
=1/2 * root (1+k^2)
Make a substitution of tanC
= 1/2 * integration of sec^3 x
Integration by parts and done ( don't forget to sub EVERYTHING back in)
is that the same x as before? If so you went in a huge circle. Where did a k come from?? (I'm actually confused - trying to work it out)
asianese
Σ
- Joined
- Sep 20, 2010
- Messages
- 2,219
- Gender
- Undisclosed
- HSC
- 2012
Re: MX2 Integration Marathon
2 calls for an expansion of the denominator which results in 1-2sin2x by identities and double angles. Then a t=tanx substitution is made, which falls (pretty sure) into a perfect square on the bottom, leading to a linear power integral.
2 calls for an expansion of the denominator which results in 1-2sin2x by identities and double angles. Then a t=tanx substitution is made, which falls (pretty sure) into a perfect square on the bottom, leading to a linear power integral.
seanieg89
Well-Known Member
- Joined
- Aug 8, 2006
- Messages
- 2,653
- Gender
- Male
- HSC
- 2007
Re: MX2 Integration Marathon
:=\int_0^1\frac{x^{s-1}-1}{\log(x)}\,dx=\int_{-\infty}^0 \frac{e^{sx}-e^x}{x}\,dx$ for real $s\geq 1$. \\$G(1)$ is trivially $0$. Now for $s\geq 1$ we have:\\ $G'(s)=\int_{-\infty}^0\frac{\partial }{\partial s}\left(\frac{e^{sx}-e^x}{x}\right) \,dx=s^{-1}$ (by differentiating under the integral).\\So $G(s)=G(1)+\int_1^s t^{-1}\, dt=\log(s)$ for all $s\geq 1$. $)
Found a pretty slick way of doing this.new question:
find
} \, \mathrm{d}x, \quad n>0)
Last edited:
Shadowdude
Cult of Personality
- Joined
- Sep 19, 2009
- Messages
- 12,141
- Gender
- Male
- HSC
- 2010
Re: MX2 Integration Marathon
what is this
leibniz rule?
what is this
leibniz rule?
seanieg89
Well-Known Member
- Joined
- Aug 8, 2006
- Messages
- 2,653
- Gender
- Male
- HSC
- 2007
Re: MX2 Integration Marathon
There are many ways of justifying differentiating under the integral. Just use one that is general enough that it applies . The one I use pretty much all the time is based on the dominated convergence theorem and suffices in most real-world applications.
. The one I use pretty much all the time is based on the dominated convergence theorem and suffices in most real-world applications.
There are many ways of justifying differentiating under the integral. Just use one that is general enough that it applies
barbernator
Active Member
- Joined
- Sep 13, 2010
- Messages
- 1,435
- Gender
- Male
- HSC
- 2012
Re: MX2 Integration Marathon
the second one becomes a sec integration.
EDIT: no it doesnt
the second one becomes a sec integration.
EDIT: no it doesnt
Last edited:
seanieg89
Well-Known Member
- Joined
- Aug 8, 2006
- Messages
- 2,653
- Gender
- Male
- HSC
- 2007
Re: MX2 Integration Marathon
Yeah, the initial substitution of mine was unnecessary of course. Just something I like doing, prefer to work with exponential functions rather than logarithmic ones.
good job
i did it a similar way:
etc.
It follows that I = G(n-1) = ln(n).
Also, asianese try and do the second one without t-results
seanieg89
Well-Known Member
- Joined
- Aug 8, 2006
- Messages
- 2,653
- Gender
- Male
- HSC
- 2007
Re: MX2 Integration Marathon
Easier.the second one becomes a sec integration.
EDIT: no it doesnt
nightweaver066
Well-Known Member
- Joined
- Jul 7, 2010
- Messages
- 1,585
- Gender
- Male
- HSC
- 2012
Re: MX2 Integration Marathon
I think it becomes the standard sec^2 and sec2xtan2x
Cbb with paper lol
Talking about the second one rolpsy posted?the second one becomes a sec integration.
EDIT: no it doesnt
I think it becomes the standard sec^2 and sec2xtan2x
Cbb with paper lol
bleakarcher
Active Member
- Joined
- Jul 8, 2011
- Messages
- 1,503
- Gender
- Male
- HSC
- 2013
Re: MX2 Integration Marathon
Now it's easy.
2/[cos^2(x)-2sin(x)cos(x)+sin^2(x)]=2sec^2(x)/[1-2tan(x)+tan^2(x)]=2sec^2(x)/[tan(x)-1]^2Talking about the second one rolpsy posted?
I think it becomes the standard sec^2 and sec2xtan2x
Cbb with paper lol
Now it's easy.
D94
New Member
- Joined
- Oct 5, 2011
- Messages
- 4,419
- Gender
- Male
- HSC
- N/A
Re: MX2 Integration Marathon
Alternatively, you could straightaway divide the top and bottom by cos2x.2/[cos^2(x)-2sin(x)cos(x)+sin^2(x)]=2sec^2(x)/[1-2tan(x)+tan^2(x)]=2sec^2(x)/[tan(x)-1]^2
Now it's easy.
bleakarcher
Active Member
- Joined
- Jul 8, 2011
- Messages
- 1,503
- Gender
- Male
- HSC
- 2013
Re: MX2 Integration Marathon
Yeah I guess that saves expanding [cos(x)-sin(x)]^2 haha.Alternatively, you could straightaway divide the top and bottom by cos2x.
- Status
- Not open for further replies.
