Re: 2012 HSC MX2 Marathon
New question: Prove by induction that every natural number greater than 2 has a prime divisor.
There is a stronger result, that every natural number greater than 1 has a prime divisor. Anyhow, several methods, first as per the question states:
Assume a set

where
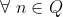
, then n > 1 and n has a prime divisor. Now to show that k exists in Q is quite straightforward with the following partition:
If k has no other factors than k or 1, then k is trivially in Q.
If k is nonprime, then k will have a factor w such that
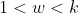
, thus
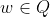
by the inductive hypothesis.
hence

Next method is to show a even stronger result, in other words, the fundmental theorem of arithmetic.
Assume that there are numbers which can not be expressed as a product of primes. Let the smallest possible number of this kind be

.

can not be
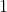
since
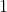
is neither composite nor prime.

can not be prime since the PPF of a prime number is just itself. Thus

must be a composite number.
Let the composition of

where

Since

was the smallest number that can not be expressed as a product of primes, this means

and

can be expressed as a product of primes and consequently we get

where

and

can be both expressed as primes. Contradiction!
Thus

can also be expressed as a product of primes.
Lemma 1: If
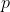
is a prime and
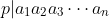
then
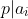
for some
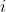
.
Lemma 2: If
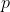
and
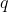
are primes and

is a natural number and
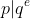
then
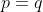
.
Let's assume that for some number

that there are
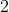
(at least) ways of expressing its PPF.

Clearly for all
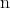
,


By
Lemma 1 
for any

.
By
Lemma 2 
This means that for all
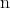
and all

there are values of
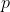
which equals to those of
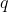
. For example,
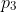
could equal to
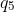
, or
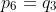
etc. This also means we have created a bijection between
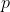
and
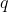
such that
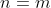
.
Therefore if the number

has
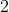
PPF's then the prime number 'base' will be exactly the same, the only different would be in the powers, namely
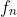
and
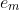
.
Now since each
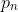
has a corespondent equivalent
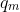
we can rewrite

as:


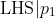
however
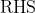
can not be divided by
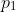
unless for some

such that

But since
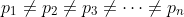
we have a contradiction.
