MX2 Integration Marathon (3 Viewers)

Qeru · Feb 4, 2021

vernburn said:
It does look like it doesn’t it! Jkjk
In reality, I really only like integration now because there is a certain elegance to it and skill required (and it’s still fresh in my head). I find the rest of maths quite boring and stale imho. My attempts at the above integrals are really just due to boredom (not long till uni starts now though).

Doesn't look like you were bored of maths considering you got a 99 for 4U. There had to be some interest there lol

CM_Tutor · Feb 4, 2021

Qeru said:
The ultimate tedious integral:

$\int \sqrt[5]{\tan{x}} dx$

This can easily be converted to a much more recognisable integral with the substitution:

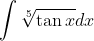
$\begin{align*} \text{Let:} \qquad u^5 &= \tan^2{x} \\ \text{Implicitly differentiating with respect to $x$:} \qquad 5u^4\frac{du}{dx} &= 2\tan{x} \times \sec^2{x} \\ &= 2\sqrt{\tan^2{x}}(\tan^2{x} + 1) \\ &= 2\sqrt{u^5}(u^5+ 1) \\ \frac{5u^4}{2u^2\sqrt{u}(u^5 + 1)}du &= dx \\ dx &= \frac{5u^2}{2\sqrt{u}(u^5 + 1)}du \end{align*}$

From which it follows that:

$\begin{align*} I &= \int{\sqrt[5]{\tan{x}}}\ dx \\ &= \int{\left(u^{\frac{5}{2}}\right)^{\frac{1}{5}} \times \frac{5u^2}{2\sqrt{u}(u^5 + 1)}}\ du \qquad \text{where $u^5=\tan^2{x}$} \\ &= \int{\sqrt{u} \times \frac{5u^2}{2\sqrt{u}(u^5 + 1)}}\ du \\ &= \frac{5}{2}\int{\frac{u^2}{u^5 + 1}}\ du \end{align*}$

and the problem is reduced to a very (very) messy partial fractions problem. According to the integral calculator, and after cleaning up its awful formatting / presentation, the indefinite integral is:

$I = \int{\sqrt[5]{\tan{x}}}\ dx$

$= \frac{\sqrt{5}-1}{8}\ln\left[2\sqrt[5]{\tan^4{x}}-\left(\sqrt{5}-1\right)\sqrt[5]{\tan^2{x}}+2\right] + \frac{\sqrt{10+2\sqrt{5}}}{4}\tan^{-1}\left[\frac{4\sqrt[5]{\tan^2{x}}-\sqrt{5}-1}{\sqrt{10-2\sqrt{5}}}\right]$

$- \left(\frac{\sqrt{5}+1}{8}\ln\left[2\sqrt[5]{\tan^4{x}}+\left(\sqrt{5}-1\right)\sqrt[5]{\tan^2{x}}+2\right] + \frac{\sqrt{10-2\sqrt{5}}}{4}\tan^{-1}\left[\frac{4\sqrt[5]{\tan^2{x}}+\sqrt{5}-1}{\sqrt{10+2\sqrt{5}}}\right]\right)$

$+ \frac{1}{2}\ln\left(\sqrt[5]{\tan^2{x}}+1\right)+C \qquad \text{for some constant $C$}$

stupid_girl · Feb 4, 2021

I wrote this in the past but I have now forgotten how to solve it.
$\int_{0}^{1}\log_{2}\left(\frac{\left(16^{\sqrt{x}}+k\cdot4^{\sqrt{x}}+4\right)\left(16^{1-\sqrt{x}}+k\cdot4^{1-\sqrt{x}}+4\right)}{\left(4^{\sqrt{x}}+k\cdot2^{\sqrt{x}}+4\right)\left(4^{1-\sqrt{x}}+k\cdot2^{1-\sqrt{x}}+4\right)}\right)dx=1$

Edit: I remember how to solve it now.

CM_Tutor · Feb 6, 2021

stupid_girl said:
I wrote this in the past but I have now forgotten how to solve it.
$\int_{0}^{1}\log_{2}\left(\frac{\left(16^{\sqrt{x}}+k\cdot4^{\sqrt{x}}+4\right)\left(16^{1-\sqrt{x}}+k\cdot4^{1-\sqrt{x}}+4\right)}{\left(4^{\sqrt{x}}+k\cdot2^{\sqrt{x}}+4\right)\left(4^{1-\sqrt{x}}+k\cdot2^{1-\sqrt{x}}+4\right)}\right)dx=1$

Is the question here to prove this result - i.e. that the integral is 1 irrespective of the value of k - or is this an equation that needs to be solved to find the value(s) of k?

stupid_girl · Feb 6, 2021

CM_Tutor said:
Is the question here to prove this result - i.e. that the integral is 1 irrespective of the value of k - or is this an equation that needs to be solved to find the value(s) of k?

The integral is 1 irrespective of the value of k.

s97127 · Feb 8, 2021

stupid_girl said:
I wrote this in the past but I have now forgotten how to solve it.
$\int_{0}^{1}\log_{2}\left(\frac{\left(16^{\sqrt{x}}+k\cdot4^{\sqrt{x}}+4\right)\left(16^{1-\sqrt{x}}+k\cdot4^{1-\sqrt{x}}+4\right)}{\left(4^{\sqrt{x}}+k\cdot2^{\sqrt{x}}+4\right)\left(4^{1-\sqrt{x}}+k\cdot2^{1-\sqrt{x}}+4\right)}\right)dx=1$

Edit: I remember how to solve it now.

can you post the solution here? This gives me a headache for the last few days

Qeru · Feb 8, 2021

s97127 said:
can you post the solution here? This gives me a headache for the last few days

Yeah lol, some factoring trick I imagine, or King Rule but I dont see how that is used.

stupid_girl · Feb 12, 2021

s97127 said:
can you post the solution here? This gives me a headache for the last few days

What tricks have you tried?

The following techniques are NOT required.

integration by parts
differentiation under the integral sign
hyperbolic function

s97127 · Feb 15, 2021

stupid_girl said:
What tricks have you tried?

The following techniques are NOT required.
integration by parts
differentiation under the integral sign
hyperbolic function

I've tried all the techniques above. I think the problem will be solved by substituting x with another variable t and we got I + I = 2 so I = 1.
Am i on the right path?

stupid_girl · Feb 15, 2021

By adjusting the coefficient of k to 1, you should be able to take out log_2 (2), which is just 1. Integrating dx from 0 to 1 gives you 1.

The remaining factors have the same structure and you can offset them by suitable substitution.

idkkdi · Feb 15, 2021

stupid_girl said:
By adjusting the coefficient of k to 1, you should be able to take out log_2 (2), which is just 1. Integrating dx from 0 to 1 gives you 1.

The remaining factors have the same structure and you can offset them by suitable substitution.

if ur stupid, what am i.

F

Paradoxica · Feb 16, 2021

Define

$\psi(a,k,u,v) := \log_a\left(a^{u} + k + a^{v}\right)$

The original question takes the argument a=2.

For brevity, we will suppress the first two arguments⁰, as it turns out they are irrelevant to the final answer.

(This is a thing in higher mathematics lmao get used to it)

The original question is equivalent¹ to:

$\int_0^1 \left(\psi\left(2\sqrt{x},2-2\sqrt{x}\right)+\psi\left(2-2\sqrt{x},2\sqrt{x}\right)- \psi\left(\sqrt{x},2-\sqrt{x}\right) - \psi\left(1-\sqrt{x},1+\sqrt{x}\right) + \nolinebreak 1 \right) \mathrm{d}x$

It is clear that ψ(u,v) = ψ(v,u), from the definition, so we can collapse the first two terms together.

Break off the +1 term and ignore it for now, as that is what gives the final answer.

Using the substitution x = t², the integral becomes:

$2 \int_0^1 \left(2\psi\left(2t,2-2t\right) - \psi\left(t,2-t\right) - \psi\left(1+t,1-t\right) \right) t \mathrm{d}t$

Using the boundary preserving transformation t→1-t, this becomes:

$2 \int_0^1 \left(2\psi\left(2-2t,2t\right) - \psi\left(1-t,1+t\right) - \psi\left(2-t,t\right) \right) (1-t) \mathrm{d}t$

Use symmetry in u,v, once again, to reorder the arguments to be the same as before.

Take the average of this and the previous step, to obtain:

$\int_0^1 \left(2\psi\left(2t,2-2t\right) - \psi\left(t,2-t\right) - \psi\left(1+t,1-t\right) \right) \mathrm{d}t$

We consider only the last two terms for now.

Define:

$A := \int_0^1 \psi\left(t,2-t\right) \mathrm{d}t; \quad B := \int_0^1 \psi\left(1+t,1-t\right) \mathrm{d}t$

By the same boundary preserving transformation as before, it is obvious that A = B.

Use the scaling transformation t→2t:

$A = B = 2\int_0^{\frac{1}{2}} \psi\left(2t,2-2t\right) \mathrm{d}t$

Use the same "boundary preserving transformation" to change the interval [0,½] → [½,1]

$B = 2\int_{\frac{1}{2}}^1 \psi\left(2-2t,2t\right) \mathrm{d}t = 2\int_{\frac{1}{2}}^1 \psi\left(2t,2-2t\right) \mathrm{d}t$

Finally, we have:

$A + B = 2\int_0^1 \psi\left(2t,2-2t\right) \mathrm{d}t = \int_0^1 2\psi\left(2t,2-2t\right) \mathrm{d}t$

Returning to the step before we defined A and B, we obtain:

$\int_0^1 2\psi\left(2t,2-2t\right) \mathrm{d}t -(A+B) = \int_0^1 2\psi\left(2t,2-2t\right)-2\psi\left(2t,2-2t\right) \mathrm{d}t = 0$

The +1 term is trivially integrated to obtain 1, which is the final answer.

⁰ ψ(u,v) = ψ(a,k,u,v)

¹ left as an exercise to the reader

Paradoxica · Feb 16, 2021

the real answer to this conundrum is that by using a substitution which maps a finite open interval to ℝ, you cannot invert the substitution to obtain an antiderivative valid over all of ℝ. the substitution is only bijective locally, not globally, so a true inverse does not exist, hence the antiderivative is only valid over specific subsets of ℝ. in order to obtain an antiderivative that is valid on all of ℝ, you need a different method.

more to read here:

https://dl.acm.org/doi/pdf/10.1145/174603.174409

https://www.maa.org/sites/default/files/pdf/cms_upload/0025570x43754.di021186.02p0083t.pdf

https://core.ac.uk/download/pdf/82517224.pdf

stupid_girl · Apr 6, 2021

Qeru · Apr 17, 2021

stupid_girl said:
$\int_{1}^{100}\frac{x^{3}-x\cos^{2}\left(2\ln x\right)}{x^{4}+x^{2}\sin\left(4\ln x\right)+\cos^{2}\left(2\ln x\right)}dx=\frac{11\pi}{8}+\frac{1}{2}\tan^{-1}\left(\frac{1+10000\tan\left(4\ln10\right)}{10000}\right)$

Let $u=\tan^{-1}\left(\frac{1+x^2\tan{(2\ln{x})}}{x^2}\right)$

From derivative calc:
$\frac{du}{dx}=\frac{4x^3\sec^2(2\ln x)-2x}{x^4\tan^2(2\ln x)+2x^2\tan(2\ln x)+x^4+1}$

$\frac{du}{dx}=\frac{4x^3-2x\cos^2(2\ln x)}{x^4\sin^2(2\ln x)+2x^2\sin(2\ln x)\cos(2\ln x)+(x^4+1)\cos^2(2\ln x)}$

$\frac{du}{dx}=\frac{4x^3-2x\cos^2(2\ln x)}{x^4+x^2\sin(4\ln x)+\cos^2(2\ln x)}$

$I=\int_{1}^{100}\frac{x^{3}-x\cos^{2}\left(2\ln x\right)}{x^{4}+x^{2}\sin\left(4\ln x\right)+\cos^{2}\left(2\ln x\right)}dx$

$I=\int_{1}^{100}\frac{2x^{3}-x\cos^{2}\left(2\ln x\right)}{x^{4}+x^{2}\sin\left(4\ln x\right)+\cos^{2}\left(2\ln x\right)}dx-\int_{1}^{100}\frac{x^{3}}{x^{4}+x^{2}\sin\left(4\ln x\right)+\cos^{2}\left(2\ln x\right)}dx$

$I=\frac{1}{2}\int_{1}^{100}1du-\int_{1}^{100}\frac{x^{3}}{x^{4}+x^{2}\sin\left(4\ln x\right)+\cos^{2}\left(2\ln x\right)}dx$

$I=\frac{1}{2}\tan^{-1}\left(\frac{1+10000\tan\left(4\ln10\right)}{10000}\right)-\frac{\pi}{8}-\int_{1}^{100}\frac{x^{3}}{x^{4}+x^{2}\sin\left(4\ln x\right)+\cos^{2}\left(2\ln x\right)}dx$

No clue how to do the second integral.

stupid_girl · Apr 17, 2021

Qeru said:
Let $u=\tan^{-1}\left(\frac{1+x^2\tan{(2\ln{x})}}{x^2}\right)$

From derivative calc:
$\frac{du}{dx}=\frac{4x^3\sec^2(2\ln x)-2x}{x^4\tan^2(2\ln x)+2x^2\tan(2\ln x)+x^4+1}$

$\frac{du}{dx}=\frac{4x^3-2x\cos^2(2\ln x)}{x^4\sin^2(2\ln x)+2x^2\sin(2\ln x)\cos(2\ln x)+(x^4+1)\cos^2(2\ln x)}$

$\frac{du}{dx}=\frac{4x^3-2x\cos^2(2\ln x)}{x^4+x^2\sin(4\ln x)+\cos^2(2\ln x)}$

$I=\int_{1}^{100}\frac{x^{3}-x\cos^{2}\left(2\ln x\right)}{x^{4}+x^{2}\sin\left(4\ln x\right)+\cos^{2}\left(2\ln x\right)}dx$

$I=\int_{1}^{100}\frac{2x^{3}-x\cos^{2}\left(2\ln x\right)}{x^{4}+x^{2}\sin\left(4\ln x\right)+\cos^{2}\left(2\ln x\right)}dx-\int_{1}^{100}\frac{x^{3}}{x^{4}+x^{2}\sin\left(4\ln x\right)+\cos^{2}\left(2\ln x\right)}dx$

$I=\frac{1}{2}\int_{1}^{100}1du-\int_{1}^{100}\frac{x^{3}}{x^{4}+x^{2}\sin\left(4\ln x\right)+\cos^{2}\left(2\ln x\right)}dx$

$I=\frac{1}{2}\tan^{-1}\left(\frac{1+10000\tan\left(4\ln10\right)}{10000}\right)-\frac{\pi}{8}-\int_{1}^{100}\frac{x^{3}}{x^{4}+x^{2}\sin\left(4\ln x\right)+\cos^{2}\left(2\ln x\right)}dx$

No clue how to do the second integral.

You choose the correct substitution but calculate du/dx incorrectly.
$\frac{du}{dx}=\frac{2x^3-2x\cos^2(2\ln x)}{x^4+x^2\sin(4\ln x)+\cos^2(2\ln x)}$

stupid_girl · Apr 17, 2021

By the way, choosing the correct substitution is not the end of story.

Remember to split the interval.
$\int_{1}^{100}\frac{x^{3}-x\cos^{2}\left(2\ln x\right)}{x^{4}+x^{2}\sin\left(4\ln x\right)+\cos^{2}\left(2\ln x\right)}dx=\int_{1}^{e^{\frac{\pi}{4}}}\frac{x^{3}-x\cos^{2}\left(2\ln x\right)}{x^{4}+x^{2}\sin\left(4\ln x\right)+\cos^{2}\left(2\ln x\right)}dx+\int_{e^{\frac{\pi}{4}}}^{e^{\frac{3\pi}{4}}}\frac{x^{3}-x\cos^{2}\left(2\ln x\right)}{x^{4}+x^{2}\sin\left(4\ln x\right)+\cos^{2}\left(2\ln x\right)}dx+\int_{e^{\frac{3\pi}{4}}}^{e^{\frac{5\pi}{4}}}\frac{x^{3}-x\cos^{2}\left(2\ln x\right)}{x^{4}+x^{2}\sin\left(4\ln x\right)+\cos^{2}\left(2\ln x\right)}dx+\int_{e^{\frac{5\pi}{4}}}^{100}\frac{x^{3}-x\cos^{2}\left(2\ln x\right)}{x^{4}+x^{2}\sin\left(4\ln x\right)+\cos^{2}\left(2\ln x\right)}dx$

If you are wondering how these break points are chosen, you may try to graph $u=\tan^{-1}\left(\frac{1+x^2\tan{(2\ln{x})}}{x^2}\right)$ .

stupid_girl · Apr 18, 2021

stupid_girl · Apr 25, 2021

stupid_girl · May 22, 2021

MX2 Integration Marathon (3 Viewers)

Well-Known Member

Moderator

Active Member

Moderator

Active Member

Active Member

Well-Known Member

Active Member

Active Member

Active Member

Well-Known Member

-insert title here-

-insert title here-

Active Member

Well-Known Member

Active Member

Active Member

Active Member

Active Member

Active Member

Users Who Are Viewing This Thread (Users: 0, Guests: 3)